Compounding can produce astounding results. Take the Berkshire Hathaway Annual Letter that Warren Buffett writes. His latest shows a 20.3% annual compound return between 1965 and 2019. Impressive, especially versus the S&P500 return of 10%.
But here’s the punchline. That 20.3% is a 2,744,062% overall gain. Or put another way, $100 invested with Buffett for 55 years would now be worth $2.75 million.
An unwritten rule for every article about compounding is that the author must state that Albert Einstein described compound interest as “the eighth wonder of the world”.
Trouble is, despite the internet carrying thousands of memes on the phrase, there is no evidence he ever said it. Economist Don Stammer once told me he had spent days trying to find an original and accurate source of the quote without success. Never mind, fake news, but it sounds good.
Einstein’s reaction when asked for the 800th time about this quote

Although he never said it, it’s true
You can see why the quote is so popular. It’s the single most powerful concept to understand and experiment with to appreciate investing and long-term financial planning.
Now, I realise if I start quoting mathematical formulas, many readers will fall into two camps:
- The ‘smarties’ (actuaries, I’m looking at you) who will wonder if there is any person on the planet who does not already know this. Let me remind them that 50% of people don’t know what 50% means.
- The ‘impatients’ who have far too much to do than think about a mathematical formula and are more interested in watching the next episode of The Last Dance.
So for the record, I’ll squeeze a simple compounding formula here which assumes one interest payment a year. FV = P(1 + r)^n where FV is the future value, P is the current principal, r is the interest rate (expressed as a decimal such that 5% is 0.05) and n is the number of periods.
It’s an elegantly simple concept. Choose any interest rate to compound any amount over any number of future periods to see how much it will be worth. An easy way to do this is to use the function on Excel to play around, as described in four minutes in this video. I promise this is an easy skill you will treasure for the rest of your life.
Let’s check some results
Firstlinks recently published an article on why future returns are likely to be worse than in the past. While many superannuation fund calculators still assume a 7.5% (or 5% real) return, leading researchers now argue real returns of around 2% are more likely.
This change has profound implications for investor outcomes and behaviour.
Obviously, the higher the rate of return, the more spectacular the compounding. According to data from Shane Oliver at AMP Capital (see chart below) the return since 1926 for Australian equities is 10.9% and residential real estate 10.7%.
Table 1 below compounds returns over various periods using four real return assumptions:
- 7.5% real (or 10% nominal), an extremely optimistic outlook similar to past returns
- 5% real, which is still optimistic with rates so low
- 2.5% real, perhaps from a balanced portfolio with good exposure to growth assets
- 0% real or about 2.5% nominal, which is an optimistic assumption for anyone stuck in term deposits or cash.
The results from investing $100,000 at the start are as follows:
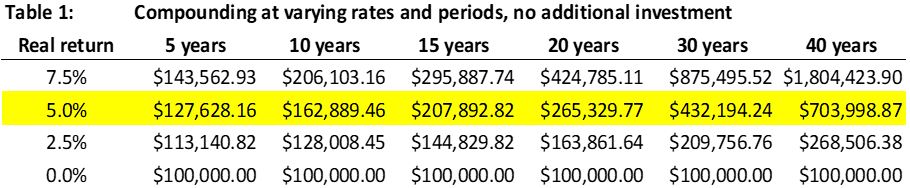
Let’s focus for a moment on the shaded 5% results (assumes interest compounded at 5%):
- At the end of five years, the principal has grown to $127,628. The extra $2,628 over the simple interest of $25,000 is interest on interest. Doesn’t sound like much.
- At the end of 10 years, principal has reached $162,889. Now there is $12,889 added to the simple interest of $50,000. Both are periods of five years, one with an extra $2,628, the other with $12,889. This is compounding, interest on interest, kicking in.
- After 20 years, the principal reaches $265,330, an increase of $165,330 over the initial principal of $100,000. The increase in years 10 to 20 was $102,440, even more than the initial principal, and a big increase on the rise in the first 10 years of $62,889.
- After 30 years, the balance has reached $432,194 and after 40 years, the original $100,000 has reached $704,000, or seven times the original principal.
Even Einstein, if he had ever thought about it, would consider this a miracle.
If we use 7.5% real for 40 years, which investors from previous generations could have achieved just by buying residential property or an index fund, $100,000 would be worth $1.8 million.
And for, say, a family endowment fund which is run over many generations, investing $100 in 1926 at 10.9% grows to over $1.6 million over 94 years. All you need to do is live long enough and stay invested.
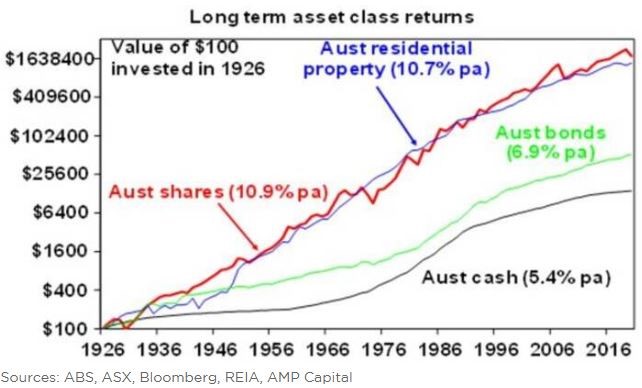
Table 2 shows how quickly the interest on interest dominates the interest on the original principal. Consider how much the value of the investments increases in each decade over a 40-year period.
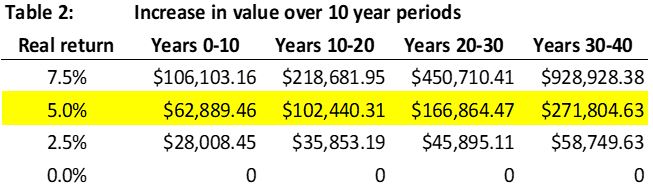
At the heady rate of 7.5%, the final decade alone grows by nearly a million dollars.
What investing lessons do these numbers teach us?
Every person is different and will aspire to varying standards of living and spending ability in retirement. Based on the ASFA Retirement Standards, a couple will need $62,435 a year for a comfortable retirement (and this assumes they also own their home and pay no rent).
For the sake of this analysis, let’s say someone inherits $100,000 at the age of 25 and wants $1 million by the time they reach 65.
Now we can see the powerful lessons behind these numbers:
- If they can achieve a 7.5% real return, they will have $1.8 million without saving anything else (except paying off their home and covering any tax). If $1 million is sufficient, they might retire early, reaching this amount after 32 years. As this amount is below the $1.6 million pension cap, all earnings will be tax free after age of 60 or 65. In fact, a couple can have up to $3.2 million in a tax-free pension.
- If they earn 5% real, after 40 years they would have $700,000. This is still an outstanding result but to reach the $1 million, they will need to sit down with a financial adviser and decide an extra savings plan.
- If they earn 2.5% real, at the end of 40 years, they will have only $268,000. The amount is falling dramatically, reaching only one-quarter of their goal. Now the couple needs a significant extra savings plan which may involve working longer.
- If they leave their money in cash or term deposits earning the inflation rate (a circumstance facing a vast number of retirees now), then at best the purchasing power of their $100,000 is retained. It will do little to finance a comfortable retirement.
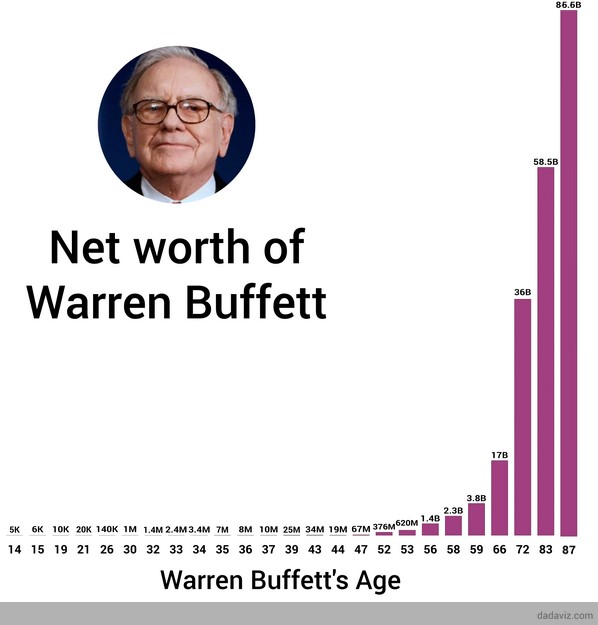
This is a massive issue for today’s investors. In the past, wealth could be accumulated over time by relying on high compounding returns. That’s why many older ‘average’ income earners live in multi-million dollar homes. Back on the example of Warren Buffett, the following chart estimates how much his wealth has compounded in later decades. It shows that 99% of his wealth came after the age of 50. It’s not that the percentage return was better, or that Berkshire Hathaway pays him dividends, which it doesn’t. It’s the compounding impact. In fact, he has donated a lot of money to charities in his later years.
You don’t need to be wealthy to become a millionaire. You need time and good investment performance. For example, start with nothing and invest $5,000 a year at 10% for 32 years and the amount will reach $1 million.
Low returns require a fundamental rethink. Far more of the retirement nest egg will need to come from savings over a longer period to avoid missing a lifestyle goal.
Old people with a lot of wealth are not usually brilliant investors. They are just old, and they have allowed their assets to compound over long periods in a favourable environment. On average in Australia at the moment, 60-year-old men have $270,000 in superannuation. At 5% real, assuming no tax and no money drawn out (yes, I know there are minimum drawdowns on a pension account, but it doesn't need to be spent), it will grow to $1.2 million by the age of 90. If a man dies a millionaire, don’t begrudge the wealth.
The Rules of 72, 114 and 144
Of course, all this is linked to the Rule of 72. Dividing 72 by the annual rate of interest gives an estimate of how long it takes to double an investment. Put 7.2% into your Excel calculator for 10 years with $100,000 and the result is $200,400. Near enough double.
But what about the Rule of 114, which tells how long it takes for an investment to triple (at 10%, about 11 years) and the Rule of 144, when an investment quadruples (at 10%, about 14.5 years).
Crucially, going from 72 to 144 does not go from double to triple: it quadruples! That’s compounding.
Other implications: fees and borrowings
The impact of fees on investing is reinforced by studying compounding. An active manager must really justify their fees, not deliver index-like performance for active fees. In itself, 1% does not sound like much, but compounded over an investing lifetime the result is dramatic.
For example, $100,000 invested at 5% real for 30 years grows to $432,000. Now impose a 1% fee and earn 4%, and the final amount falls to $324,000, or $108,000 less. That’s a drop of 25% for a 1% fee. Impose a 2% fee and earn only 3%, the final amount drops to $243,000, a fall of 44%.
And all this good stuff on investing works in reverse for borrowing. Remember the other side of the phrase that Einstein never said: “He who understands it, earns it … he who doesn’t, pays it.” For most people, repayment of non-tax-deductible borrowings is their best investment.
In this ‘save early, save often, save for a long time’ message, the last word goes to Buffett, and it applies for most people, even those of average means.
“I always knew I was going to be rich, so I was never in a hurry.”
Graham Hand is Managing Editor of Firstlinks. This article is general information and does not consider the circumstances of any investor.